Crystal oscillators: bringing synchronization to digital electronics

By Qin Zhuang, Applications Engineering Manager, Diodes Incorporated

The majority of electronics systems encountered at home or work will comprise at least one oscillator working as a clock that delivers accurate timing, synchronizes operations or acts as a frequency reference. Here, we’ll be looking at the many benefits of using quartz crystal oscillators and discussing the choices available.

There are numerous assorted clock signals within microprocessor-based systems, used for implementing instructions, delivering external communication interfaces and moving data to and from the memory.

While the clock frequency of a straightforward embedded controller might be around a few megahertz, that of a PC is usually expected to have a clock input of 15MHz, which is then multiplied within to deliver clocks for the CPU and other subsystems. Additional components found in the systems might well have their own clock requirements such as a 25MHz clock for an Ethernet controller or the 32.768kHz required for a real-time clock (RTC).

Furthermore, a precise frequency reference is a prerequisite for a radio-frequency (RF) system in order to facilitate end-to-end communication as well as ensuring unwelcome noise and signals are filtered out.

Main oscillator features

In addition to delivering the specified frequency, an oscillator may have to meet further needs, subject to the nature of the application. For instance, several applications call for an accurately defined frequency, which is especially significant for systems required to communicate with other devices through wireless or serial interfaces. Accuracy like this is usually measured as parts per million (ppm).

Low-power consumption is crucial in battery-powered or handheld appliances and is particularly pertinent for an RTC, being in a constantly active state, even in standby and low-power modes.

Lastly, considerations such as cost, form factor and operating environment will need to be determined.

Perfect pitch

Every oscillator employs a type of resonant or tuned circuit, alongside amplification and feedback, to produce an output at a particular frequency.

A tuned circuit can be created on an inductance-capacitance (LC) or resistance-capacitance (RC) network, both being simple assemblies that permit the frequency to be altered across a wide range. However, creating an accurate LC or RC oscillator involves using expensive precision components; and even then, they do not always meet the highest levels of precision and stability needed by most applications.

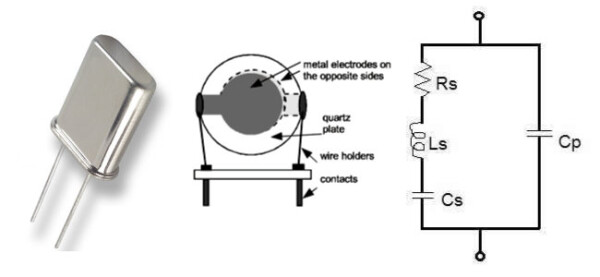
The crystal resonator is made of quartz, the extreme physical stability of which makes it the first choice as a resonant component. It is cut with two parallel faces which have metal contacts positioned onto them. Quartz displays a piezoelectric effect, meaning that when placed under pressure, a voltage is produced across the faces. Likewise, an applied voltage causes the crystal to change shape in a shear move.

In addition, feedback makes the crystal oscillate at its natural resonant frequency, which is determined by the size of the crystal and how it is cut. The most common cut form is called AT and can be deployed across a wide range of frequencies as well as demonstrating good thermal stability.

With a very high quality (Q) factor, crystal resonators demonstrate a highly accurate and extremely stable frequency, which means that they can be applied as the base product for very precise, low-cost oscillators.

Crystal Structure and Corresponding Circuit

The construction of a crystal resonator and its corresponding circuit is shown in Figure 1, where the value Cp denotes the capacitance of the two parallel electrodes. Components Rs, Ls and Cs, represent the crystal’s mechanical properties of internal friction, mass and elasticity.

The corresponding circuit demonstrates there are two possible resonant frequencies: one owing to the series connection of Ls and Cs, and the second where Cp is in parallel with the inductance. This series resonance is defined by:

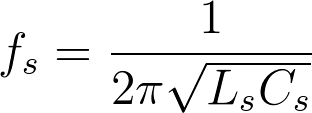
Series Resonance Formula

 The parallel resonant frequency is expressed as:

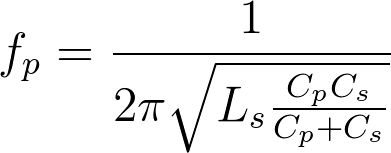
Parallel Resonant Frequency Formula

These two frequencies are usually less than 1% apart, with the oscillator circuit defining which one of the resonant modes is to be employed. In the event, the majority of oscillators utilize the parallel mode.

For higher frequencies, above the 75MHz mark, the crystal is capable of oscillating at a multiple of the fundamental frequency, or overtone.

Oscillator circuits

An oscillator circuit is often incorporated into a device that needs a reference clock signal. For instance, numerous microcontrollers, and comparable applications, comprise two pins to which you can straightforwardly connect a crystal product, together with a pair of ceramic capacitors to complete the oscillation circuit.

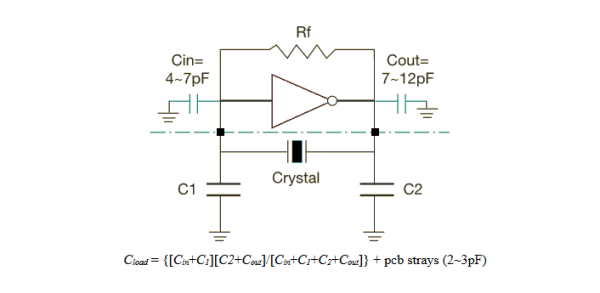
Oscillator Components and Stray Capacitance

The circuit’s total load capacitance (CL) must meet the specified CL of the crystal. This comprises the ceramic capacitors combined with any stray capacitance derived from the crystal package, the oscillator’s input pins and the circuit board tracks.

Since it is quite tricky to calculate accurately the combined stray and parasitic capacitances within the circuit, try starting with an estimate (usually around 4 to 6pF) and then measure the output frequency to establish whether the value of the capacitors requires adjustment.

However, if the total CL is larger than the specified CL, then the oscillation frequency will be reduced. Equally, where the CL is too low, the frequency will be higher; and if the CL is too far out in either direction, then the oscillator might not start up at all.

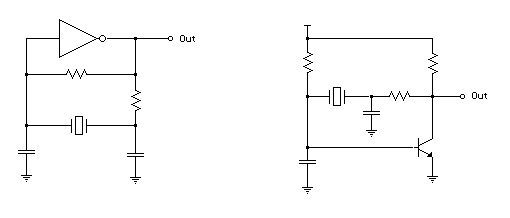
Figure 3. Oscillator Circuits

The diagram above shows an external oscillator circuit built using a transistor or inverting logic gate as the feedback amplifier. Since designing a high-quality oscillator is a tough challenge, even when most crystal retailers supply design guides, it might be easier to buy an off-the-shelf oscillator module. Such oscillator modules comprise a crystal and all the components required, including the load capacitors, guaranteeing you a cost-effective, high-performance oscillator. All that’s down to you is in providing an appropriate power supply.

Figure 4. Crystal Oscillator Module

Crystal oscillator modules are a good option for applications requiring precise and reliable frequencies, like wireless communications systems or Ethernet interfaces. Diodes Inc. manufactures all kinds of crystal oscillator products in assorted size and ppm accuracy to suit different applications.  

Variations on a theme

The fact that the frequency of an external CL fluctuates opens up the possibility of building a crystal oscillator where the output can be tweaked within a small range. This proves handy in RF applications where a receiver has to fine-tune its own frequency to correspond to the received signal.

Voltage-controlled crystal oscillators (VCXOs) employ devices called varactors (or varicap diodes) as their load capacitors. The varactors’ capacitance alters with an applied control voltage which subsequently changes the frequency of oscillation.

The main limitations of a VCXO are ‘pullability’, clock jitter and control voltage range: 

• ‘Pullability’ outlines the frequency change for any given change in control voltage; with a larger value indicating that the oscillator can work over a larger range, but a smaller value resulting in better stability and reduced phase noise. The maximum tuning range is typically around the +/-200ppm mark.
• Clock jitter occurs at higher than a fixed-frequency oscillator, especially at the outer extremes of the tuning range where it’s working at its limits.
• Control voltage is normally from 0V to 2 or 3V.

When you need greater stability, for example less than 10ppm, over working temperature range than can be found in a normal crystal oscillator, you need to select a temperature-compensated crystal oscillator (TCXO). Like the standard oscillator module mentioned earlier, these are also available as off-the-shelf modules and come with a wide range of parameters.

A TCXO contains a circuit that is able to measure the ambient temperature and then generate a control voltage to modify the frequency of a VCXO in order to counteract the effect of temperature changes. The TCXO calculates the control voltage needed from the crystal’s temperature-frequency response curve.

In addition, a TCXO module regularly incorporates its own voltage regulator, safeguarding the oscillator against variations in the external supply voltage.

Conclusions

As a resonant component, quartz crystal delivers a very precise, stable and inexpensive frequency reference. Furthermore, crystals and crystal oscillators now come with an impressive range of parameters and applications to meet the requirements of your design.

What’s more, numerous devices integrate the oscillator circuitry, facilitating further the design-in process. If it’s a higher-quality clock you require, crystal oscillator modules can provide an effective alternative, demonstrating higher precision and better stability than their integrated oscillator equivalents; and remember, these modules are also available with voltage-controlled frequency or temperature compensation.